Workshop on New Trends in Mathematical Biology
Time: Saturday, October 29, 2022
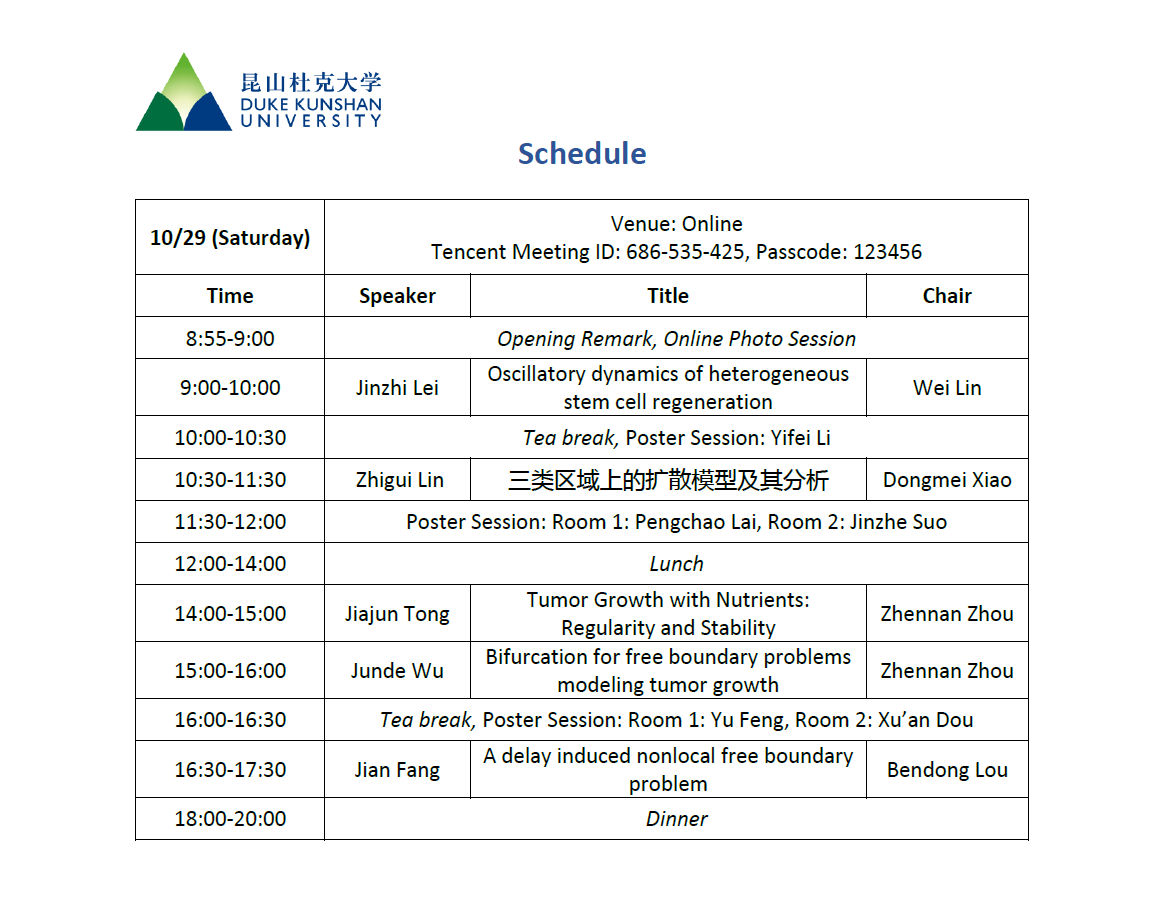
Venue: Online, Tencent Meeting ID: 686-535-425, Passcode: 123456
Organizers:
Yuan Lou, Shanghai Jiao Tong University
Yu Feng, Peking University
Xiaoqian Xu, Duke Kunshan University
Zhennan Zhou, Peking University
Schedule:
Titles and Abstracts:
Oscillatory dynamics of heterogeneous stem cell regeneration
Jinzhi Lei
Tiangong University
Stem cell regeneration is an essential biological process in the maintenance of tissue homeostasis; dysregulation of stem cell regeneration may result in dynamic diseases that show oscillations in cell numbers. Cell heterogeneity and plasticity are necessary for the dynamic equilibrium of tissue homeostasis; however, how these features may affect the oscillatory dynamics of the stem cell regeneration process remains poorly understood. Here, based on a mathematical model of heterogeneous stem cell regeneration that includes cell heterogeneity and random transition of epigenetic states, we study the conditions to have oscillation solutions through bifurcation analysis and numerical simulations. Our results show various model system dynamics with changes in different parameters associated with kinetic rates, cellular heterogeneity, and plasticity. We show that introducing heterogeneity and plasticity to cells can result in oscillation dynamics, as we have seen in the homogeneous stem cell regeneration system. However, increasing the cell heterogeneity and plasticity intends to maintain tissue homeostasis under certain conditions. The current study is an initiatory investigation of how cell heterogeneity and plasticity may affect stem cell regeneration dynamics, and many questions remain further studied biologically and mathematically.
三类区域上的扩散模型及其分析
林支桂
Yangzhou University
物种入侵是目前三大环境问题之一。 本报告首先介绍固定区域上的种群扩散问题,接着考虑变化区域上的种群扩散。区域的演化分为两种:已知的和未知的。 前者通常是环境引起,后者是种群自身发展的要求。对于周期演化区域上的Logistic种群扩散,给出基本再生数,得到演化率对种群扩散的影响;而对于自由变化区域上的Logistic种群扩散问,定义与时间有关的生态再生指标, 给出扩张-灭绝二择一结果和扩张时的渐近速度。然后介绍自由变化区域上的SIS传染病模型,给出时空风险指标;最后介绍描述新冠病毒的有向图上的扩散。
Tumor Growth with Nutrients: Regularity and Stability
Jiajun Tong
Peking University
In this talk, we discuss a tumor growth model with nutrients. The model admits dynamic patch solutions due to the contact inhibition among the tumor cells. When the nutrients do not diffuse and the cells do not die, we show that the tumor density exhibits regularizing dynamics. In particular, we provide contraction estimates, exponential rate of asymptotic convergence, and boundary regularity of the tumor patch. These results are in sharp contrast to the models either with nutrient diffusion or with non-zero death rate in tumor cells, where complex behavior such as dendritic growth may arise. This talk is based on a joint work with Matt Jacobs and Inwon Kim.
Bifurcation for free boundary problems modeling tumor growth
Junde Wu
Soochow Technology
In this talk we consider several free boundary problems modeling the growth of nonnecrotic and necrotic tumor spheroids, which are originated from the ideas of H. P. Greenspan, H. M. Byrne and M. J. Chaplain. The models consist of a diffusion equation for the nutrient and an elliptic equation for the pressure, and the tumor surface is a moving boundary. Nonnecrotic tumor models have been extensively studied and many illuminative results have been established. We shall mainly discuss bifurcation analysis of the necrotic tumor model based on the Crandall-Rabinowitz theorem, and recent progress on this topic.
A delay induced nonlocal free boundary problem
Jian Fang
Harbin Institute of Technology
Incorporating time delay and Stefan type free boundary into reaction-diffusion equation yields a nonlocal problem. Under a KPP type setting we establish a dichotomy on propagation or vanishing. When propagation happens, the spreading speed is shown to exist and it is determined nonlinearly by a delay-induced nonlocal elliptic problem in half line. This talk is based on a joint work with Yihong Du and Ningkui Sun.
Schedule, titles and abstracts to be downloaded here: New Trends in Mathematical Biology -Duke Kunshan University.
Special thanks to the NSFC fund and the Research Support Office at Duke Kunshan University.
